Novel Range Wise Optimization of the Exponential Bounds on the Gaussian $Q$ Function and its Applications in Communications Theory
Keywords:
communication systems, approximate computing, optimization algorithms, bit error rate, performance evaluationAbstract
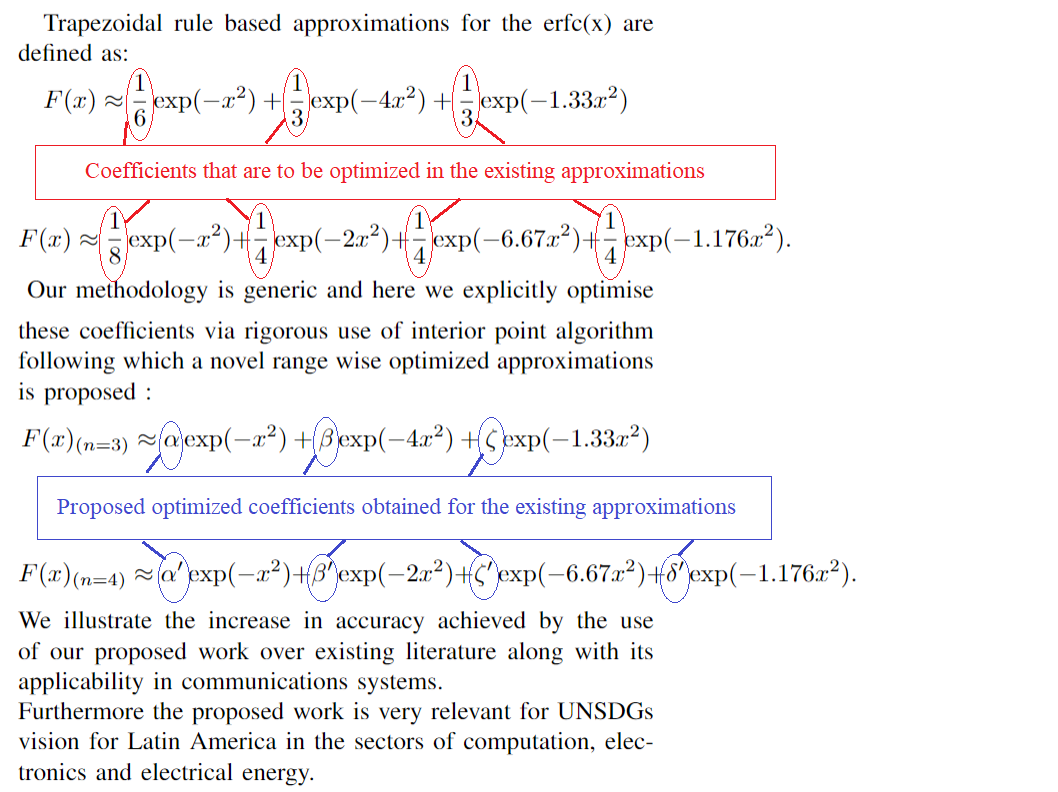
This paper presents a novel and highly effective method for improving the accuracy of approximations for the Gaussian $Q$ function. By rigorously optimizing the coefficients of the approximations using the interior point optimization technique, significantly tighter bounds are achieved with simplicity intact. The proposed approach, which is applicable to a wide range of scenarios, focuses on enhancing the simple exponential bounds proposed in the literature. Through a comprehensive analysis based on the relative error, the superiority of the optimized coefficients compared to the existing bounds and approximations available in the open literature is demonstrated. Moreover, an insight into the generic applicability of the optimized coefficients is provided, which exhibits excellent performance in terms of the absolute error as well. The Gaussian $Q$ function plays a crucial role in evaluating the performance of diverse wireless communication systems under various challenging fading distributions. Therefore, the proposed research significantly contributes to advancing the accuracy of the approximations of the Gaussian $Q$ function, enabling improved error performance for coherent digital modulation techniques. The findings presented herein offer valuable contributions to the state-of-the-art and set a new standard for accuracy in the work related to Gaussian $Q$ function approximations.
Downloads
References
M. K. Simon and M.-S. Alouini, Digital Communication over Fading Channels, 2nd ed. Wiley, 2005.
M. Ramirez and S. Molano, “Analytical expression for the bit error rate
of a filtered multitone modulation scheme (FMT) through a multipath
fading channel,” IEEE Latin America Transactions, vol. 19, no. 4, pp.
–651, Apr. 2021.
S. Aggarwal, “Approximate solution to SEP integral over Fluctuating
Beckmann fading,” Wireless Personal Communications, vol. 111, pp.
–1376, 2020.
D. N. and R. B., “Performance analysis of wireless powered sensor
network with opportunistic scheduling over generalized κ−μ shadowed
fading channels,” Physical Communication, vol. 53, p. 101727, 2022.
M. Bilim, “Cascaded double fading channels: A case of κ − μ shadow-
ing,” Physical Communication, vol. 52, p. 101649, 2022.
G. Llano Ramirez, J. Reig, and L. Rubio, “Modeling and analysis in
frequency of the UWB channel with lognormal statistics for MB-OFDM
systems,” IEEE Latin America Transactions, vol. 7, no. 1, pp. 33–41,
Mar. 2009.
M. Chiani, D. Dardari, and M. K. Simon, “New exponential bounds
and approximations for the computation of error probability in fading
channels,” IEEE Trans. Wireless Commun., vol. 2, no. 4, pp. 840–845,
Jul. 2003.
G. K. Karagiannidis and A. S. Lioumpas, “An improved approximation
for the Gaussian Q-function,” IEEE Commun. Lett., vol. 11, no. 8, pp.
–646, Aug. 2007.
Y. Isukapalli and B. Rao, “An analytically tractable approximation for
the Gaussian Q-function,” IEEE Commun. Lett., vol. 12, no. 9, pp. 669–
, Sep. 2008.
P. Loskot and N. C. Beaulieu, “Prony and polynomial approximations for
evaluation of the average probability of error over slow-fading channels,”
IEEE Trans. Veh. Technol., vol. 58, no. 3, pp. 1269–1280, Mar. 2009.
W. M. Jang, “A simple upper bound of the Gaussian Q-function with
closed-form error bound,” IEEE Commun. Lett., vol. 15, no. 2, pp. 157–
, Feb. 2011.
M. L ́opez-Ben ́ıtez and F. Casadevall, “Versatile, accurate, and analyti-
cally tractable approximation for the Gaussian Q-function,” IEEE Trans.
Commun., vol. 59, no. 4, pp. 917–922, Apr. 2011.
Q. Shi and Y. Karasawa, “An accurate and efficient approximation to
the Gaussian Q-function and its applications in performance analysis in
Nakagami-m fading,” IEEE Commun. Lett., vol. 15, no. 5, pp. 479–481,
May 2011.
G. Abreu, “Very simple tight bounds on the Q-function,” IEEE Trans.
Commun., vol. 60, no. 9, pp. 2415–2420, Sep. 2012.
O. Olabiyi and A. Annamalai, “Invertible exponential-type approxima-
tions for the Gaussian probability integral Q(x) with applications,” IEEE
Wireless Commun. Lett., vol. 1, no. 5, pp. 544–547, Oct. 2012.
Y. Mouchtak and F. E. Bouanani, “New tighter upper bounds on the per-
formance of convolutional code over exponentially correlated Rayleigh
fading channel,” in Proc. IEEE International Wireless Commun. and
Mobile Computing Conf. (IWCMC), Sep. 2016.
D. Sadhwani, R. N. Yadav, and S. Aggarwal, “Tighter bounds on
the Gaussian Q function and its application in Nakagami−m fading
channel,” IEEE Wireless Commun. Lett., vol. 6, no. 5, pp. 574–577,
Oct. 2017.
F. E. Bouanani, Y. Mouchtak, and G. K. Karagiannidis, “New tight
bounds for the Gaussian Q-function and applications,” IEEE Access,
vol. 8, pp. 145 037–145 055, Aug. 2020.
I. M. Tanash and T. Riihonen, “Global minimax approximations and
bounds for the Gaussian Q-function by sums of exponentials,” IEEE
Trans. Commun., vol. 68, no. 10, pp. 6514–6524, Oct. 2020.
——, “Quadrature-based exponential-type approximations for the Gaus-
sian Q-function,” in Proc. IEEE 93rd Vehicular Technol. Conf.
(VTC2021-Spring), Apr. 2021.
——, “Improved coefficients for the Karagiannidis–Lioumpas approxi-
mations and bounds to the Gaussian Q-function,” IEEE Commun. Lett.,
vol. 25, no. 5, pp. 1468–1471, May 2021.
S. Aggarwal, “Average of product of two Gaussian Q functions as
summation series and its significance in evaluating SEP integrals over
fading channels,” Journal of the Franklin Institute, vol. 138, no. 15, pp.
–8060, Oct. 2021.
D. Sadhwani, A. Powari, and N. Mehta, “New, simple and accurate
approximation for the Gaussian Q function with applications,” IEEE
Commun. Lett., vol. 26, no. 3, pp. 518–522, Mar. 2022.
A. Powari and D. Sadhwani, “Extremely accurate, generic and tractable
exponential bounds for the Gaussian Q function with applications in
communication systems,” IEEE Commun. Lett., vol. 27, no. 7, pp. 1724–
, Jul. 2023.
G. Astfalk, I. Lustig, R. Marsten, and D. Shanno, “The interior-point
method for linear programming,” IEEE Software, vol. 9, no. 4, pp. 61–
, 1992.
S.-J. Kim, K. Koh, M. Lustig, S. Boyd, and D. Gorinevsky, “An interior-
point method for large-scale ℓ1-regularized least squares,” IEEE Journal
of Selected Topics in Signal Processing, vol. 1, no. 4, pp. 606–617, 2007.
C.-N. Lu and M. Unum, “Network constrained security control using an
interior point algorithm,” IEEE Transactions on Power Systems, vol. 8,
no. 3, pp. 1068–1076, 1993.
J. Momoh and J. Zhu, “Improved interior point method for OPF
problems,” IEEE Transactions on Power Systems, vol. 14, no. 3, pp.
–1120, 1999.
P. Zhao and S. Yu, “An improved interior point algorithm for quantile
regression,” IEEE Access, vol. 8, pp. 139 647–139 657, 2020.
M. I. Khan and K. Xia, “Effective self adaptive multiple source local-
ization technique by primal dual interior point method in binary sensor
networks,” IEEE Communications Letters, vol. 21, no. 5, pp. 1119–1122,
A. Powari, D. Sadhwani, L. Gupta, and R. N. Yadav, “Optimization of
the exponential bounds on the Gaussian Q function using interior point
algorithm and its application in communication theory,” IEEE Open
Journal of the Communications Society, pp. 1–1, 2022.
D. Sadhwani, “Simple and tightly approximated integrals over κ-μ
shadowed fading channel with applications,” IEEE Trans. Veh. Technol.,
vol. 67, no. 10, pp. 10 092–10 096, 2018.
M. P. Ninos, H. E. Nistazakis, H. G. Sandalidis, and G. S. Tombras,
“CDMA RoFSO links with nonzero boresight pointing errors over M
turbulence channels,” IEEE Photonics Journal, vol. 10, no. 5, pp. 1–12,
Dec. 2018.
G. K. Varotsos, H. E. Nistazakis, H. Sandalidis, G. S. Tombras, and
W. Gappmair, “SIMO subcarrier PSK FSO links with phase noise
and non-zero boresight pointing errors over turbulence channels,” IET
Communications, vol. 13, no. 7, pp. 831–836, 2019.
H. Ma, G. Cai, Y. Fang, J. Wen, P. Chen, and S. Akhtar, “A new
enhanced energy-detector-based FM-DCSK UWB system for tactile
internet,” IEEE Transactions on Industrial Informatics, vol. 15, no. 5,
pp. 3028–3039, 2019.
M. H. Khoshafa, T. M. N. Ngatched, M. H. Ahmed, and A. R.
Ndjiongue, “Active reconfigurable intelligent surfaces-aided wireless
communication system,” IEEE Commun. Lett., vol. 25, no. 11, pp. 3699–
, Dec. 2021.
L. Yang, J. Yang, W. Xie, M. O. Hasna, T. Tsiftsis, and M. D. Renzo,
“Secrecy performance analysis of RIS-aided wireless communication
systems,” IEEE Trans. Veh. Technol., vol. 69, no. 10, pp. 12 296–12 300,
P. Yang, L. Yang, W. C. Kuang, and S. J. Wang, “Outage performance
of cognitive radio networks with a coverage-limited RIS for interference
elimination,” IEEE Wireless Commun. Lett., pp. 1–1, 2021.
Y. Jia, Z. Wang, J. Yu, and P.-Y. Kam, “A new approach to deriving
closed-form bit error probability expressions of MPSK signals,” IEEE
Trans. Commun., pp. 1–1, 2023.
H. Lei, F. Yang, I. S. Ansari, H. Liu, K. J. Kim, and T. A. Tsiftsis,
“Secrecy outage performance analysis for uplink CR-NOMA systems
with hybrid SIC,” IEEE Internet of Things Journal, pp. 1–1, 2023.
D. Sadhwani and R. N. Yadav, “A comprehensible form of the product
of two Gaussian Q functions and its usefulness in κ-μ shadowed fading
distribution,” Journal of the Franklin Institute, Elsevier, vol. 357, pp.
–5123, Apr. 2020